James Algebra uses three distinct types of Containers to express numeric and non-numeric structure.
Figure 6-1 shows the Round Boundary, the Square Boundary, and the Angle Boundary. In Iconic Arithmetic volume 1, we’ll stick to the interpretation of James Forms in Figure 6-1.
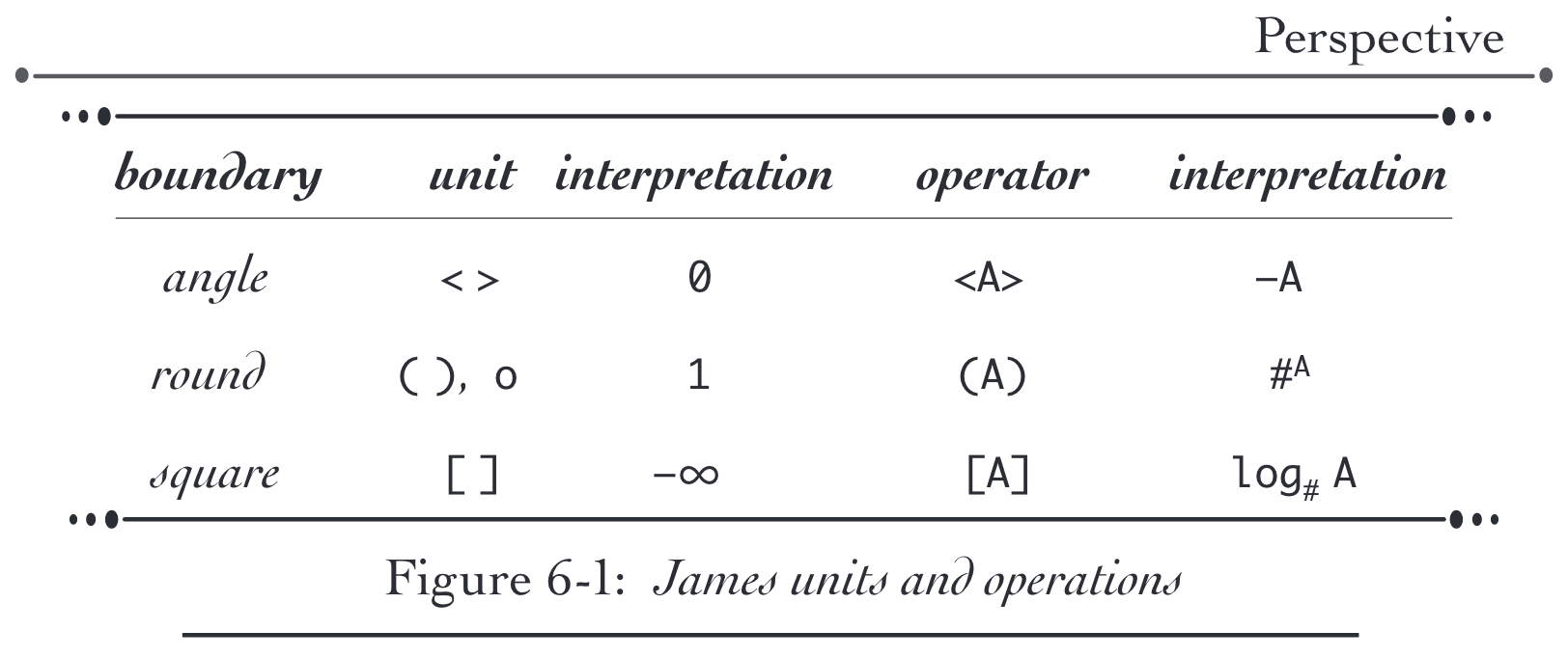
Figure 6-1: James units and operations
Multiplication-as-substitution, characteristic of unit-ensembles, will not be used in the following chapters.
Boundary forms are Icons. Pictorial forms trigger not only different conceptual models, they trigger different physiological processes. Transcription is therefore more than a cognitive shift, it implicates different perceptual systems and a different behavioral vocabulary.
The only relation within a boundary calculus is that of Containment, a minimal conceptual basis consisting of one binary relation. The Contains Relation is quite general. When expressed within logic, containment can be interpreted as implies. When expressed as a network, containment is directly-connected-to. When expressed as a set, it’s called is-a-member. When expressed as a number, it is successor. When expressed as a map, it’s shares-a-common-border. Within the context of a pile of blocks, contains becomes supported-by. When seen as a family relationship, it is parent-of. When described as an abstract mathematical structure, it is a rooted tree. All of these metaphors share a collection of common characteristics that are concretized by the properties of physical containers. The fundamental concept underlying containment is Distinction: a container distinguishes Inside from Outside.
[…]
Iconic Arithmetic Volume II looks at the structure that the binary relations contains, implies, is-a-member, successor, and parent-of have in common: each makes a distinction between Container and Contained. This chapter provides an initial discussion of the mechanisms of the James pattern algebra of distinctions.